Mortgage Payments
Overview:
Ever look at your year-end mortgage statement (Form 1098) and wonder why the interest paid during the year is almost equal to the cash you paid during the year?
Purpose:
The reason you pay so much interest as a percentage of total payments in the early years of the loan is due to the way a mortgage loan is calculated. This article will show how a loan works and why paying more towards principal in early years can save you significant total cash outflow throughout the term of the loan. {Editor’s Note: for this article we will use the word loan, as many loans and mortgages follow the similar payment method.}
Assumptions:
Let’s lay out how a loan payment schedule works. We’ll assume that monthly payments throughout the loan will be the same as is with most car, home & business loans. First, we’ll need to calculate the monthly payments. You can back into the number using some other calculations, but an easier way is to calculate the monthly payment amount is by using Excel and the PMT function. So, let’s lay out some assumptions we’ll use in our calculations:
- Loan term will be 10 years or 120 months
- Annual Interest rate will be 6% or .5% monthly
- Original loan amount will be $100,000
Using the above assumptions and the PMT function in Excel we get a monthly payment of $1,110.21.
Analysis # 1:
So, let’s what through how the loan payment schedule (also called an amortization schedule) works with the assumptions we made above.
At the beginning of month 1, the loan has a balance of $100,000. At the end of the first month, we need to calculation interest on the loan, so we take the loan balance of $100,000 and owe interest of $500 ($100,000 * .5%) for month 1. The different between the monthly payment of $1,110.21 and the interest of $500 is $610.21 and is our principal paydown for month 1. At the end of the first month, the new ending loan balance is $99,389.79 (calculated as $100,000 minus the $610.21 principal paydown).
At the beginning of month 2, the loan balance is $99,389.79. At the end of the second month, we take the loan balance of $99,389.79 and owe interest of $496.95 ($99,389.79 * .5%) for month 2. The different between the monthly payment of $1,110.21 and the interest of $496.95 is $613.26 and is our principal paydown for month 2. At the end of the second month, the new ending loan balance is $98,776.53 (calculated as $99,389.79 minus the $613.26 principal paydown). And so on, until the principal balance gets to $0.
Some things to notice about just the first 2 months:
- The interest charge is very large as a percentage of the total payment. For month 1, we paid $500 of interest on a payment of $1,110.21, thus our payment is 45% interest and only 55% principal. However, by month 2, the interest percentage drops to 44% and 56% to principal.
- The monthly payment stays the same.
- Between month 1 and 2 the interest charge per month goes
- Between month 1 and 2 the principal charge per month goes
This will continue throughout the loan, with the % of interest dropping close to zero by the last months of the loan. This means that early in our loan we are mostly paying interest of the loan and nothing towards principle. Below is a chart of the monthly interest and principal amounts throughout the loan.
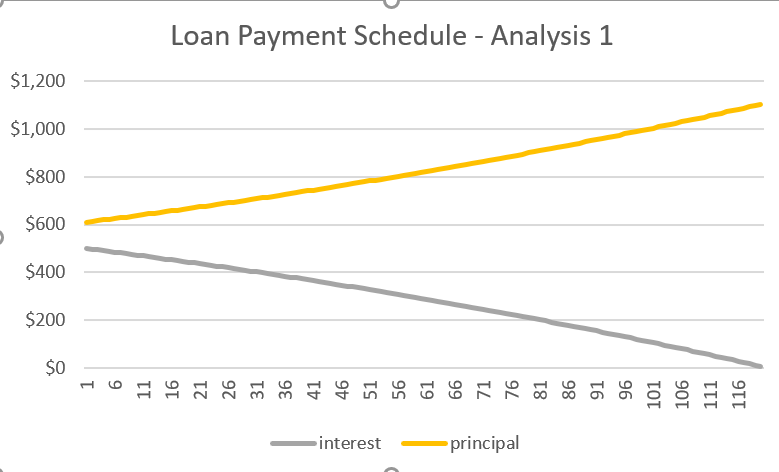
Analysis # 2:
Over the course of this loan, you’ll pay $33,224.38 of interest on a principal of $100,000. Thus, you are paying more than 33% of the original loan balance just in interest. Your total cash outflow for this loan over 10 years will be $133,224.38. There can be a way to limit the amount of interest you pay, by paying additional principal early.
Let’s use the same assumption above but say during month 1 you make an additional principal payment of $10,000, which is an opportunity cost in that you could be using that cash for investing or paying off other debt. So now the updated ending balance of $89,389.79.
At the beginning of month 2, the loan balance is $89,389.79. At the end of the second month, we take the loan balance of $89,389.79 and owe interest of $446.95 ($89,389.79 * .5%) for month 2. The different between the monthly payment of $1,110.21 and the interest of $496.95 is $663.26 and is our principal paydown for month 2. At the end of the second month, the new ending loan balance is $88,726.53 (calculated as $89,389.79 minus the $663.26 principal paydown).
Notice compared to Analysis # 1 – for month 2, we’ve paid $50 less of interest and $50 more towards our principal. While this is a small amount for just for one payment, by the end of the loan the difference can really add up.
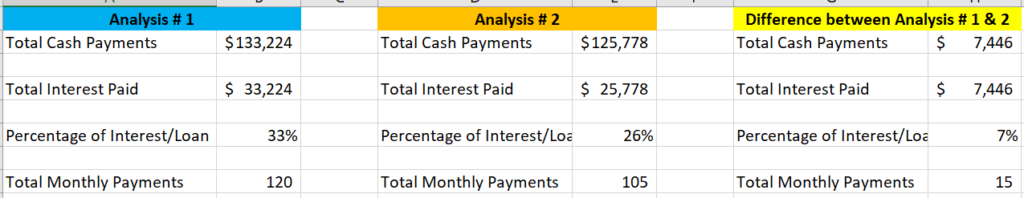
Noted that by paying that $10,000 you save over $7,446.11 in interest and have 15 less monthly payments. Below is a chart of the monthly interest and payment amounts throughout the loan.
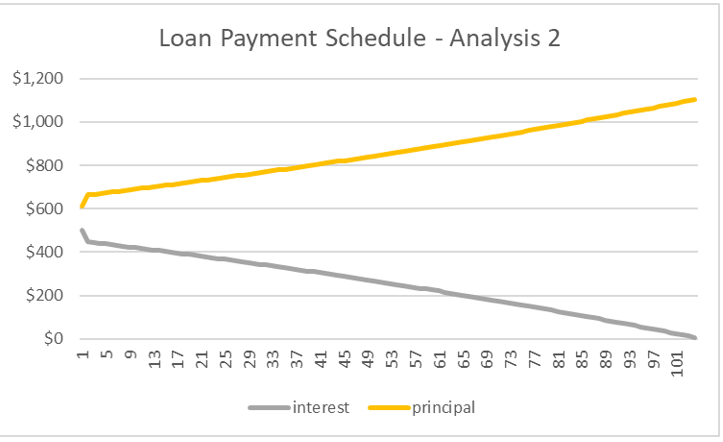
Conclusion:
As we’ve walked through above, the early portions of a loan are significantly weighted towards interest vs. principal payment. Making extra payments that go towards principal will help decrease the total amount of cash flow outflow on a loan. There are some tax advantages to the mortgage loan interest, however for many loans there is no tax advantage. As always, discuss with your tax advisor before making any decisions that might alter your tax positions. You can use this excel file, which I love to use to help me understand what an additional principal payment will save me in interest overall.
Action Plans:
- Review through your loans and see what any additional principal payment would save you in total cash outflow;
- Review if additional principal payments might be better than your opportunity cost, say of investing by a 401(k) contribution; and
- Review our articles on Finance First on interest rates and see if getting a lower interest rate on a loan (though maybe with a fee) would lead to a decrease total cash outflow.
